Consider all planar sets A with constant width 1. Namely, in every direction, the distance between the two parallel lines that touch A from both sides is 1. We already know that there exists such sets other than the circle of radius 1/2.
Among all these sets which one has the largest perimeter? the smallest perimeter?
Combinatorics and more 
The length of a curve is proportional to the expected number of its crossings with a uniformly random line. Each constant-width curve has the same expected number of crossings — for any slope of the random line, it meets the lines with that slope in an interval of the same length — so they all have the same perimeter.
Strictly speaking, Eppstein’s argument is false, given that Gil asked about planar *sets*. Take, for instance, an annulus of outer radius 1 and inner radius 1/2. The perimeter is certainly larger than for the disk of radius 1.
David’s answer is right and beautiful but it deserves to be elaborated a little more.
The maximum perimeter is infinity if you don’t ask the figure to be convex. I guess for convex figures the infimum is assumed and independent of the figure, like in David Eppstein’s guess. To prove that I would “rotate the figure inside the 2 surfaces” and write some differential equation to control that, like in the proof of Kepler’s law.
Ok, fine, here’s a somewhat more detailed explanation.
One may define a measure on the space of lines in the plane that is invariant under rotation and translation; one way to do this is to parametrize a line by the pair (ρ,θ) where θ is the angle of the line and ρ is its (signed) distance from the origin, and use the uniform measure of points on a plane with Cartesian (not polar!) coordinates (ρ,θ). I think I first learned about this measure in a 1986 message to sci.math by William Thurston, but of course it goes back much farther than that and is um, well-known among the people who know this sort of thing. For convenience, let’s call the plane where the lines live “primal” and the plane having (ρ,θ) as Cartesian coordinates “dual”.
This measure is obviously rotation invariant (a rotation of the primal plane is just a vertical translation in the dual plane). It’s also translation invariant: a translation of the primal plane causes the ρ coordinates of all lines with angle θ to shift by the same amount, so any set in the dual plane is transformed into another set with the same measure in each horizontal slice, and by Cavalieri’s principle the whole set the same measure.
For a unit-length line segment pq, let L(pq) denote the set of lines that intersect pq. It follows from translation and rotation invariance that the measure of L(pq) is a constant independent of how pq is placed in the plane, and we may as well normalize the measure so that this constant is 1. It then follows by a limiting argument that, for any curve C, the multiset L(C) of lines that intersect C (with multiplicity equal to the number of intersection points) has measure equal to the length of C.
If C is the boundary of a constant-width curve with unit width, then in the dual (ρ,θ) plane the dual points corresponding to lines in L(C) form a set whose horizontal slices form length-1 intervals, the same as for a unit circle. The endpoints of each of these horizontal slices have multiplicity 1 in L(C) and the interior points have multiplicity 2, the same as for a circle again. By Cavalieri’s principle again, L(C) has the same measure as L(circle), so C has the same length as the circle.
In my earlier comment I used probability rather than measure: to define a uniform probability on the set of lines one has to restrict them to a subset of bounded measure, say the lines intersecting a disk big enough to contain all constant-width curves. A nice way to generate uniformly random lines that cross this disk is to pick two uniformly random points on its boundary circle and form the line connecting them.
GK: Many thanks David!
Here’s the variant of the proof that occurred to me: fix a “basepoint” inside the shape and parameterize the boundary in polar co-ordinates: say for each angle![\theta \in [0,2\pi]](https://s0.wp.com/latex.php?latex=%5Ctheta+%5Cin+%5B0%2C2%5Cpi%5D&bg=ffffff&fg=333333&s=0&c=20201002)
the boundary is at distance 
. Assuming the boundary is piecewise reasonably smooth, the length of the boundary is given by $
$. Finally, use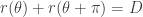 
(the fixed diamater) to see that the integral is 
.
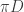By the way, doing the same calculation in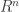
(integrating 
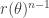over the unit sphere) seems to indicate that in general the surface area of bodies of constant width would not be constant.
Pingback: Buffon’s Needle and the Perimeter of Planar Sets of Constant Width « Combinatorics and more
Lior,
does not compute the length of the boundary. See http://en.wikipedia.org/wiki/Arclength#Finding_arc_lengths_by_integrating for a correct formula.
Another proof: (A-A)/2 is a centrally symmetric figure with constant width 1 and with the same perimeter as A. The only centrally symmetric figure with constant width is the circle, so all sets A have the same diameter.
Dear Omar, what I see at once is that (A-A)/2 is centrally symmetric. I do not see the other two two properties…
Dear Gil,
1) The width of the Minkowski sum of two planar convex figures in a certain direction is the sum of the width of the two figures in that direction. In fact, the extreme point(s) of the sum in a certain direction is (are) the sum of the extreme point(s) of the two figures in that direction.
2) The perimeter of the Minkowski sum of two planar figures is the sum of the perimeters of the two figures. This is easy for polygons and follows by approximation for any convex planar figure. For two convex polygons with m and n sides, respectively, the sum has m+n sides where each of the lentgth of the sides of the two polygons occurs exactly once. Actually, some of the m+n sides may collapse to one single side in singular cases, but then the length will add up.
Dear Omar, this is a very nice proof. Thanks!
Pingback: Test Your Intuition (10): How Does “Random Noise” Look Like. « Combinatorics and more