…while we keep discussing why mathematics is possible…
The news
Adam Marcus, Dan Spielman, and Nikhil Srivastava posted a paper entitled “Interlacing Families II: Mixed Characteristic Polynomials and the Kadison-Singer Problem,” where they prove the 1959 Kadison-Singer conjecture.
(We discussed part I of “interlacing families” in this post about new Ramanujan graphs. Looks like a nice series.)
The Kadison-Singer Conjecture
The Kadison-Singer conjecture refers to a positive answer to a question posed by Kadison and Singer: “They asked ‘whether or not each pure state of 
Updates: A very nice post on the relation of the Kadison-Singer Conjecture to foundation of quantum mechanics is in this post in Bryan Roberts‘ blog Soul Physics. Here is a very nice post on the mathematics of the conjecture with ten interesting comments on the proof by Orr Shalit, and another nice post on Yemon Choi’s blog and how could I miss the very nice post on James Lee’s blog.. Nov 4, 2013: A new post with essentially the whole proof appeared on Terry tao’s blog, Real stable polynomials and the Kadison Singer Problem.
Update: A very nice blog post on the new result was written by Nikhil Srivastava on “Windows on theory.” It emphasizes the discrapancy-theoretic nature of the new result, and explains the application for partitioning graphs into expanders.
Update (Nov 29, 2015): Erica Klarreich wrote a very nice article about it on Quanta Magazine ‘Outsiders’ Crack 50-Year-Old Math Problem, and here is a link to a SIAM news article, Kadison–Singer Problem Solved By Dana Mackenzie.
The Bourgain-Tzafriri theorem and conjecture
Let me tell again (see this post about Lior, Michael, and Aryeh where I told it first) about a theorem of Bourgain and Tzafriri related to the Kadison-Singer conjecture.
Jean Bourgain and Lior Tzafriri considered the following scenario: Let 
Consider the following hypergraph 
The vertices correspond to indices ![{}[n]=\{1,2,\dots,n\}](http://l.wordpress.com/latex.php?latex=%7B%7D%5Bn%5D%3D%5C%7B1%2C2%2C%5Cdots%2Cn%5C%7D&bg=ffffff&fg=545454&s=0 "{}[n]=\{1,2,\dots,n\}")
![S \subset [n]](http://l.wordpress.com/latex.php?latex=S+%5Csubset+%5Bn%5D&bg=ffffff&fg=545454&s=0 "S \subset [n]")
Bourgain and Tzafriri showed that for every 
Moreover, they showed that for every nonnegative weights 
The “big question” is if there a real number 
![{}[n]](http://l.wordpress.com/latex.php?latex=%7B%7D%5Bn%5D&bg=ffffff&fg=545454&s=0 "{}[n]")
Additional references:
KADISON-SINGER MEETS BOURGAIN-TZAFRIRI by PETER G. CASAZZA, ROMAN VERSHYNIN, The Kadison-Singer Problem in Mathematics and Engineering: A Detailed Account pdf, and many other recent publications by Pete Casazza.
Combinatorics and more 
I don’t understand the statement of Conjecture 1.1 of the MSS paper you have linked. Is it not true that \sum_{i=1}^{m} | |^2 = |k|^2 \eta ???
Aargh latex! Try again
. i.e. how could equation (1) hold for all vectors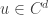 
.
It doesn’t have to hold for all vectors u. Just those of norm 1. For example,
you could achieve this if the vectors w_i are elementary unit vectors (a 1 in one
coordinate, 0 in all the others).
Thanks! I need reading glasses…
Only example I can think of is trivial:
and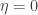 
.
If
is the union of two orthonormal bases, and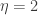 
, then (1) holds, and (2) works with 
.
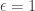BTW there is a tiny typo in Conjecture 1.1 of the paper (maybe that confused you), m should be n.
Pingback: Another one bites the dust (actually many of them) | Noncommutative Analysis
Great achievement and very clear exposition! Roughly, the main approach, which will certainly produce many more cool results and PROOFS, can be summarized as follows: associate with
a stability preserving operation OPER, possibly from some restricted class, a functional F(p) on polynomials such that there is
a bound F(OPER(p)) \geq Const(…)F(p) and iterate this bound over the products of those preservers.
The main problem to overcome: this functional F should be close(or better equal)
on “small” degree polynomials to the thing you are really interested in. If all this done then
an easy induction proof results. When I was dealing with the Van Der Waerden- Schrijver
like inequalities, the preservers are partial differentiations with zeroing and the best functional happened to be what I called the capacity. Actually,
the capacity is essentially a measure of stability. But I understood this fact after coming up with the
idea. Interestingly, the capacity was suggested by the (numerical) approach in STOC-2000 paper
with Alex Samorodnitsky(itself motivated by two Technion’s papers on the SCALING: Nemirovski-Rothblum and David London). The functional in MSS paper is what they call “Barrier Function”, in a way a “numerical’
thing as well. I envision many more algorithmic applications of the approach as well. An interesting
extension, barely touched, is to go beyond stable polynomials. For instance, the Mixed Discriminant
is related to a nice stable polynomial(the determinant) but the Mixed Volume is not. I did some
work in this direction in http://arxiv.org/abs/0812.3687(with CONJECTURES!) and http://arxiv.org/abs/cs/0702013.
Gil, your blog and https://www.facebook.com/ellina.gurvits?fref=ts are my main two WEB favorites.
Toda Roba!
Dear Leonid, many thanks –Gil
Pingback: Kadison-Singer: solved? | Since it is not ...
Pingback: Philosophy and Physics in the Kadison-Singer Conjecture | Soul Physics
It is worth point out that, not only did they solve the Weaver’s conjecture, but they solve it with optimal parameters (up to constants).
Of course, the fact that they have two sets in Corollary 1.3 (i.e. r=2) is optimal, and the bound of 1/2 + O(sqrt(alpha)) that they achieve in equation (5) is also optimal, as is shown in Section 3 of Weaver’s paper.
Gil, the statement of the original form of Kadison-Singer is a bit garbled. It should be: every pure state on an atomic maximal abelian subalgebra of B(H) has a unique extension to a pure state on B(H).
Also, +1 to Anonymous for the comment about optimal parameters. Indeed, this is the best we could have hoped for!
Thanks Nik! (I am just quoting MSS quoting KS.)
Yeah, the question they quote was something asked later by Anderson and answered negatively, assuming the continuum hypothesis, by Akemann and me. See http://www.pnas.org/content/105/14/5313.full
Actually my other comment isn’t quite accurate. The question they quote was in fact asked by Kadison and Singer, but it is not what we now know as the Kadison-Singer problem. (This other question was later sharpened by Anderson, and Akemann’s and my result falsifies both versions of the question.)
Pingback: An introduction to interlacing families | Short, Fat Matrices
Pingback: What does Kadison-Singer have to do with Quantum Mechanics? | tcs math - some mathematics of theoretical computer science
Pingback: Not mentioned recently on The Aperiodical | The Aperiodical
Tip my hat, again, to Adam,Dan and Nikhil! Let me stress why their proof looks so magically effortless and why some many experts failed before: the proof is not (random) matrix theoretic: matrices disappeared after the first application of the elementary first order differential operator,
but the proved Lax Conjecture(a hermitian solution due to Boris Dubrovin(1983) is sufficient)
allows to use (simple) matrix stuff to prove the needed convexity and monotonicity.
A similar observation applies to the comparison of A. Schrijver’s and mine proofs(no Lax though!,
but it was used in the earlier attempt http://arxiv.org/abs/math/0404474 and in STOC-2006 version).
On the other hand, in the context of the permanent, Lex proved much more in his paper
http://homepages.cwi.nl/~lex/files/countpms2.pdf and it has been heavily used in
http://arxiv.org/abs/1106.2844 to nail finally down Friedland’s conjecture on the monomer-dimer entropy(about the coefficients of the matching polynomial!).
I was thinking about possible extensions and generalizations. Of course, one natural question,
related to the description of maximizers is MSS paper, is to incorporate the ranks of A_i to
the (main) bound of Theorem 5.1. It looks like this body of work, starting with sparsifiers,
can be applied to an approximation of homogeneous real-stable polynomials, at least
multilinear ones, by polynomials with “few” variables. I noticed that the monotonicity/convexity
(Lemma 5.7) really needs just log-concavity, at least in one variable: take any log-concave function
F(t) that is
positive on [a, \infty) together with derivatives up to the third order. Then the ratio (F^{(1)}(t)/F(t))
is decreasing and convex on [a, \infty). With that in mind, I wonder about possible generalization
of the main (balancing) result of MSS to the Minkowski sums (aka zonotopes) of vector intervals \{a X_i: |a| \leq 1, X_i \in R^n\}. Of course, one will need to deal with mixed volumes instead of mixed discriminants,
the luxury of real roots and interlacing is gone, there are no known proved analogues of the Lax
conjectures…yet the Van der Waerden like bound was proved by an induction based
on the multiple partial differentiations.
Dear Leonid, this is very interesting. What is the generalization of MSS’s result to zonotopes you have in mind?
Dear Gil, roughly something of the kind:![[X_1],...,[X_m]](https://s0.wp.com/latex.php?latex=%5BX_1%5D%2C...%2C%5BX_m%5D&bg=ffffff&fg=333333&s=0&c=20201002)
and the Minkowski(volume) polynomial
![Vol(y_1[X_1]+...+y_m[X_m])](https://s0.wp.com/latex.php?latex=Vol%28y_1%5BX_1%5D%2B...%2By_m%5BX_m%5D%29&bg=ffffff&fg=333333&s=0&c=20201002)
. Let 
be the maximum coordinate of the gradient of
such that
![\sum_{i \in A}[X_i] \subset (.5 + O(\sqrt{\beta}) \sum_{1 \leq j \leq m}[X_j]](https://s0.wp.com/latex.php?latex=%5Csum_%7Bi+%5Cin+A%7D%5BX_i%5D++%5Csubset+%28.5+%2B+O%28%5Csqrt%7B%5Cbeta%7D%29+%5Csum_%7B1+%5Cleq+j+%5Cleq+m%7D%5BX_j%5D&bg=ffffff&fg=333333&s=0&c=20201002)
![\sum_{i \in B}[X_i] \subset (.5 + O(\sqrt{\beta}) \sum_{1 \leq j \leq m}[X_j]](https://s0.wp.com/latex.php?latex=%5Csum_%7Bi+%5Cin+B%7D%5BX_i%5D++%5Csubset+%28.5+%2B+O%28%5Csqrt%7B%5Cbeta%7D%29+%5Csum_%7B1+%5Cleq+j+%5Cleq+m%7D%5BX_j%5D&bg=ffffff&fg=333333&s=0&c=20201002)
.
Consider m symmetric vector intervals
the logarithm of the volume polynomial
at (1,…,1). Then there exists a partition (1,2,…,m) =
and
Needless to say, it is a very preliminary statement. Can be easily rephrased in terms of
sums of support functions.
Dear Leonid, That’s very interesting! On another matter you might be interested in the recent added comments at the end of my old post on BosonSamplin. It looks that Gaussian permanents, and perhaps even general outcomes of Boson machines are very noise-sensitive in the sense of Benjamini, Schramm and me. It would be nice to prove a general theorem of this kind (for permanents it is easy), and relate it to classical simulability perhaps via your old results on Boson machines.
Dear Gil, thanks for the pointers on noise-sensitivities. I am not very familiar with this line of research. Yet, it the context of bosons I made this observation: quantum optical distributions
are much worse concentrated than their classical approximations. To be more precise:
consider a square complex $ n \times n$ unitary matrix $U$ and associate with it two homogeneous
polynomials with non-negative coefficients:
(quantum) Q(x_1,…,x_n) = Per(UDiag(x_1,…,x_n)U^{*});
(classical) C(x_1,…,x_n) = \prod_{1 \leq i \leq n} ( \sum_{1 \leq j \leq n} |U(i,j|^2 x_j).
The sum of coefficients of both of them is 1. So they both give some prob. distributions
on integer vectors \{(r_1,…,r_n): r_1+…+r_n = n \}. Moreover, both random vectors have the same
mean, namely $(1,1,…,1)$.
Now, the coefficients of Q satisfy the
upper bound $q_{r_1,…,r_n} \leq \prod_{1 \leq i \leq n} \frac{r_i !}{r_i^{r_i}};
and the coefficients of C satisfy much smaller upper bound
$c_{r_1,…,r_n} \leq \prod_{1 \leq i \leq n} \frac{1}{r_i^{r_i}}, which is due to the simple inequality
$C(x_1,…,x_n) \leq n^{-n}(x_1+….+x_n)^n$. I do conjecture that in general
$q_{r_1,…,r_n} \leq (\prod_{1 \leq i \leq n} r_i ! ) c_{r_1,…,r_n}$.
Both bounds are sharp. Also, the distribution of $C$ can be exactly efficiently classically simulated,
even though the individual probabilities are the permanents.
Actually, the polynomial $C$ is log-concave(even stable, like those in MSS paper) on the positive orthant and Q is not.
Best, Leonid.
Dear Gil, it occurred to me that there is a connection between boson sampling and Kadison-Singer Problem:
Consider linear quantum optical distribution for $n$ bosons and $m$ detectors given by $n \times m$ “unitary” matrix $U$, i.e. $UU^{*} = I$. Let $v$ be the maximum $l_2$ norm of columns of $U$.
Let $Pr(S)$ be the probability that all bosons observed by the detectors from the subset $S$.
Then there exists a partition of detectors into two sets $[1,.,.,m] = S \cup T$ such that
$(.5)^n(1 – const v)^{n} \leq Pr(S) \leq (.5)^n(1 + const v)^{n}$ and
$(.5)^n(1 – const v)^{n} \leq Pr(T) \leq (.5)^n(1 + const v)^{n}$, where $const$ is some universal constant.
Perhaps there is a direct way to prove this, i.e. without using MSS paper.
Best, Leonid.
Dear Leonid
Many thanks for the comments. this is very interesting!
Dan Spielman is gifted!
http://www.siam.org/prizes/sponsored/polya.php
Thanks for the link, and congratulations to Adam, Dan and Nikhil!
Here is a picture with Nikhil from the Simons Institute
Nik Weaver and Charles Akemann mention that “it seems likely that the statement ‘‘every pure
state on B(H) restricts to a pure state on some atomic masa’’ is also consistent with standard set theory.” Has there been progress on this since 2008? Is anything known, e.g. under an appropriate forcing axiom? Or has CH been eliminated from the proof?
A query more than anything else…
Charles Akemann and Nik Weaver note in their 2008 PNAS paper that confirmation of the Kadison-Singer conjecture implies (S1) and (S2) are equivalent. The statement (S1) is that ‘‘every pure state on $\mathcal{B}(H)$ restricts to a pure state on some atomic masa’’, and (S2) is Anderson’s conjecture that every pure state on $\mathcal{B}(H)$ is diagonalizable, that is, of the form $f(A) =lim_{U}\langle Ae_{n}, e_{n}\rangle$ for some orthonormal basis $(e_{n})$ and some ultrafilter $U$ over $\mathbf{N}$. Prior to showing that the Continuum Hypothesis (CH) refutes (S1), they remark: “It seems likely that the statement ‘‘every pure state on $\mathcal{B}(H)$ restricts to a pure state on some atomic masa’’ is also consistent with standard set theory.”
Has there been any further progress on whether (S1) (and hence now (S2)) is relatively consistent with ZFC, e.g. under a forcing axiom? Or has CH been eliminated or weakened?
Pingback: Thanks for Additivity | Gödel's Lost Letter and P=NP
Pingback: ‘Outsiders’ Crack a 50-Year-Old Math Problem | Top News
Pingback: ‘Outsiders’ Crack a 50-Year-Old Math Problem | BTS
Pingback: ‘Outsiders’ Crack a 50-Year-Old Math Problem – Hacker Planet
Pingback: Engadget Today » ‘Outsiders’ Crack a 50-Year-Old Math Problem
Pingback: ‘Outsiders’ Crack a 50-Year-Old Math Problem | Hacker Spot
Pingback: ‘Outsiders’ Crack a 50-Year-Old Math Problem | Wiki News Tech | Tech Hub For Techgig
Pingback: 'Outsiders' Crack 50-Year-Old Math Problem | Daily Hackers News
Pingback: ‘Outsiders’ Crack a 50-Year-Old Math Problem | Gadgetleader
Pingback: ‘Outsiders’ Crack a 50-Year-Old Math Problem | News
Pingback: Thanks for Additivity | TRIFORCE STUDIO
Pingback: ‘Outsiders’ Crack a 50-Year-Old Math Problem – qwe
Pingback: ‘Outsiders’ Crack a 50-Year-Old Math Problem – BRING THE NEWS
Pingback: Solvig Kadison-Singer Problem - Skeptic Society
Pingback: Pc Scientists Resolve Kadison-Singer Thunder | Quanta Magazine | A1A
Pingback: Computer Scientists Solve Kadison-Singer Tell | Quanta Journal – Startupon.net
Pingback: Computer Scientists Solve Kadison-Singer Problem | Quanta Magazine | Digitators
Numerous colleges and universities are designed some entrance tests for explicit
topics to allow students to be certified for admission in preferential applications https://math-problem-solver.com/ .
Calculus introduces two elementary ideas which enable us to explain and examine functions.
Reblogged this on Artificial Intelligence Research Blog.